Estimating Population Size - Teacher Guide

Original Document: Estimating Population Size

Teacher Notes: You will need to prepare the populations ahead of time. You can put marbles, beads, macaroni, or other small objects in bags. The numbers will work best if the bags contain between 30 and 100 objects. I have bought toys from the dollar store for use in this project, like little plastic insects or snakes to give it more of a "biology" feel. Masking tape can serve to mark the objects, but make sure students remove the tape for the next class, extra marks will skew the data.

Another option is to use white beans you buy at the supermarket, students can use permanent markers or pens to mark the beans and then count manually at the end of the experiment. Each group could receive about a beakerful of beans to work with.

A discussion prior to this activity should address why biologists have hard time estimating populations of animals in the wild: they move around, space is too big, you cannot capture them all

1. If you were in charge of a team given the responsibility to determine the number of sunfish in Horseshoe Lake, discuss with your partner how would you accomplish this task and describe in detail below.

Answers vary, you could count the fish in a portion of the lake and then multiply by the size of the lake

 

Technique 2 - Mark and Recapture

Over the years I have collected toys to serve as sample populations, things like legos, toy soldiers, and other fake animals you can buy from

4. Calculations = Find your Population Estimate

Estimated Size - this will vary based on their data, students often forget that "10" is the number they initially marked. Some need help with equation solving and plugging numbers.

5. Use the code-name on your bag to check with the teacher about how many “animals” are really in your population.
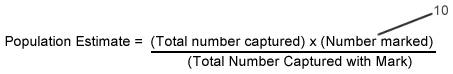
Name on Bag ___________________________ Actual Size _________ Keep this on a card for students to check, they can also count the bag themselves but I find this decreases the authenticity of the simulation. If you ask them to count, then they will say "why didn't I just do this in the first place"

This work is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License.